Monday, March 14, is Pi Day. Get it? 3/14?
Pi – that glorious and notorious number starting 3.14159265358979323846 but stretching to infinity – is incredibly important. It expresses the ratio of a circle’s circumference to its diameter. It is represented by the sixteenth letter of the Greek alphabet – π. And it goes on forever without falling into a repeating pattern (making it an irrational number).
But does it actually exist?
Weird question, I know. After all, pi is that uber-useful number that helps humans:
- Track the trajectories of objects in space.
- Model the motion of waves.
- Predict population growth.
- Determine accurate GPS navigation calculations.
- Calculate the amount of hydrogen on Jupiter.
Just to name a few things. And, our never-ending number can be arrived at easily by dividing a circular object’s circumference by its diameter.
 Or at least it can if we are measuring a perfect circle. But that is where we run into a small hitch. Where do we find a perfect circle to measure? Does one even exist in nature? According to Carnegie Mellon University . . . maybe. That’s not exactly encouraging.
Or at least it can if we are measuring a perfect circle. But that is where we run into a small hitch. Where do we find a perfect circle to measure? Does one even exist in nature? According to Carnegie Mellon University . . . maybe. That’s not exactly encouraging.
The problem is that many objects in our universe – from planets to beach balls – look like perfect circles (or spheres) to our eyes. But, when we use technology to perform precise measurements, the real truth is more bumpy, or oblong, or even a bit blobby.
As a result, when real-world measurements are plugged into the equation, the resulting number is as imperfect as real life. We get a lot of numbers that are very, very close to pi, but a perfect match is elusive. So far, no perfect circle has ever been discovered in the physical world. Even the smoothest manufactured sphere on Earth.
So, pi exists as a very useful mathematical idea, or even ideal, but you aren’t likely to find the real thing in the physical world.
But don’t take my word for it. Here are two cool activities that will teach about, celebrate, and try (but probably fail) to derive pi.
Activity 1: Can You Get Pi from Pie?
You will need:
- Circular pie
- Soft tape measure (or a piece of string and a yardstick)
- Toothpick
- Calculator
- Use the soft tape measure to measure the circumference of the pie.
 OK, so I went with my own favorite type of pie. (And, OK, I burned it too. Don’t judge.) The circumference is the distance around the outer edge, or perimeter. If you don’t have a soft tape measure, you can just use a piece of string and then measure the amount of string that was used.
OK, so I went with my own favorite type of pie. (And, OK, I burned it too. Don’t judge.) The circumference is the distance around the outer edge, or perimeter. If you don’t have a soft tape measure, you can just use a piece of string and then measure the amount of string that was used. - Record the measurement. Make sure to include your unit of measure.
My measured value was 44-5/8 inches. - Eyeball the center of the pie. Mark the spot with a toothpick.

- Measure from a point on the edge of the pie to a point on the opposite edge. Your tape measure will rest against the toothpick in the middle.

- Record the measurement.
My measured value was 14-1/4 inches. - Divide the circumference by the diameter.
If it helps, you can use a calculator to convert the fractions to decimals and solve with a calculator. For example: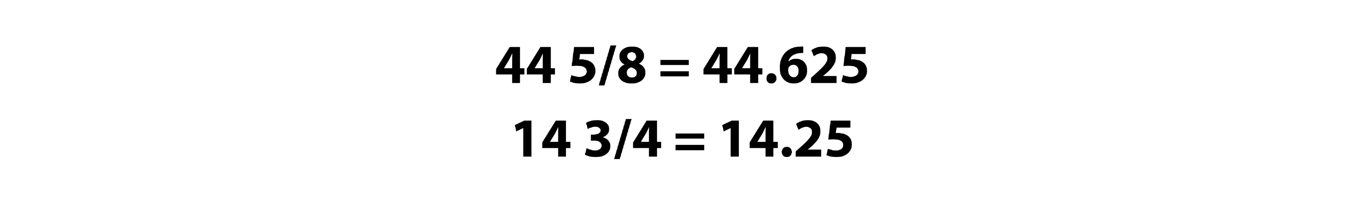 I input those numbers into my calculator:
I input those numbers into my calculator: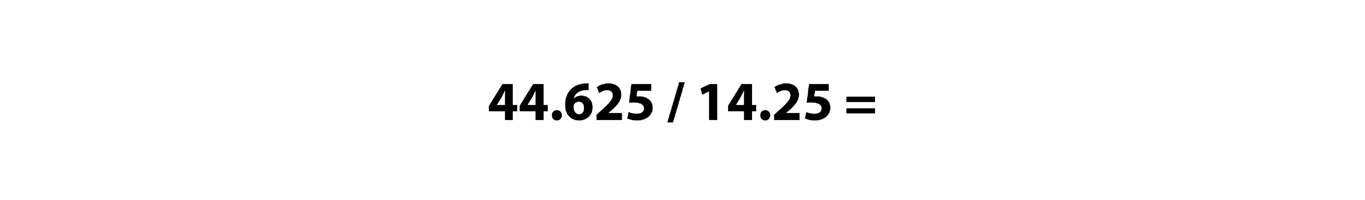 And voila!
And voila!
As you can see, it is a similar number to π, but it is not π. That is not surprising because my pie was not a truly perfect circle. I bet you will have similar findings with your result. - You can figure this step out on your own.

Activity 2: Go Outside and Measure the Planet!
Believe it or not, you can measure the distance around the Earth from your own backyard. But here’s the catch – you can only do it within a couple weeks of an equinox. For 2022, that would be:
- Spring: March 20
- Fall: September 22
Credit to the ancient Greek mathematician Eratosthenes who figured out this STEM activity two thousand years ago. We’re going to do what Eratosthenes did – estimate the Earth’s circumference by measuring a shadow. Then, we are going to do something Eratosthenes didn’t do – use that number to try to derive pi. Can we derive pi from the planet itself?
You will need:
- A sunny day at solar noon within two weeks of an equinox
- Level ground
- Yardstick (or a long straight stick or plank)
- Protractor
- String
- Small weight
- Calculator
- Use the Internet to look up: Earth’s diameter, the distance between your town and the equator, and solar noon for your location.
Here are some links that I found useful:If you can’t find your town, choose one that is near to you and use that distance. I learned that I was personally 2,588 miles from the equator.
Solar noon is the halfway point between sunrise and sunset. Bonus points if you calculate it yourself.
- Go outside a couple minutes before solar noon and set up.
- Position your yardstick vertical on flat ground.
 You can have a partner help you hold it, or you can stick it down in the ground like I did. The height doesn’t matter.
You can have a partner help you hold it, or you can stick it down in the ground like I did. The height doesn’t matter. - Make a plumb bob to ensure the stick is vertical.
 I did this on a breezy day, so you’ll have to take my word that it was vertical(ish).
I did this on a breezy day, so you’ll have to take my word that it was vertical(ish). - Mark the end point of the shadow on the ground.
 I used the scissors that I cut the string with. I could have also used a rock or a stick or my shoe.
I used the scissors that I cut the string with. I could have also used a rock or a stick or my shoe. - Connect a string between the top of the stick and the tip of the shadow.


- Use the protractor to measure the angle of the string relative to the vertical stick.
 If you think about it, you are really measuring the angle of the shadow.
If you think about it, you are really measuring the angle of the shadow. - Record the angle.
As you can see, my value was 42 degrees. - To calculate your estimate of the Earth’s circumference, use the following formula:
 Perform the operations. Round your final number to the nearest mile.
Perform the operations. Round your final number to the nearest mile.
- Use the Internet to research the actual value of Earth’s circumference. How close is your measurement?
I’m not going to give it away, but I’ll just say that my result was not a perfect hit, but it was closer than the measurement Eratosthenes arrived at. And I bet you can do even better! - Divide the circumference by Earth’s diameter. How close is your number to π?
Try it with the circumference that you calculated as well as the value you found online. Do either match π?
Pi, what a wonderful thing. And, not just the kind for eating.
Ready to explore a bit more with math? Although not pi or pie, this snack math activity, created by Chris Woods of DailySTEM, is a fun favorite.
Or, now that you’ve used the Sun to measure the planet, you’re ready to shoot for the Moon. Explore this simple moon phases activity.

 OK, so I went with my own favorite type of pie. (And, OK, I burned it too. Don’t judge.) The circumference is the distance around the outer edge, or perimeter. If you don’t have a soft tape measure, you can just use a piece of string and then measure the amount of string that was used.
OK, so I went with my own favorite type of pie. (And, OK, I burned it too. Don’t judge.) The circumference is the distance around the outer edge, or perimeter. If you don’t have a soft tape measure, you can just use a piece of string and then measure the amount of string that was used.

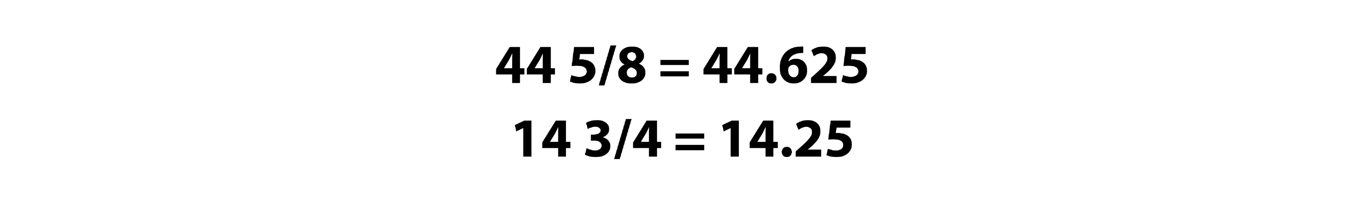 I input those numbers into my calculator:
I input those numbers into my calculator: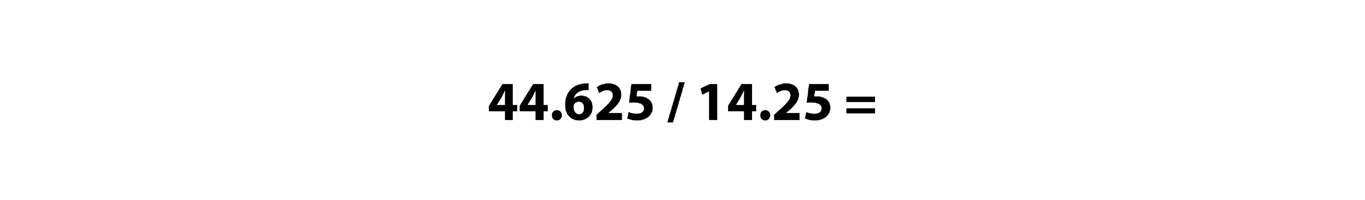 And voila!
And voila!

 You can have a partner help you hold it, or you can stick it down in the ground like I did. The height doesn’t matter.
You can have a partner help you hold it, or you can stick it down in the ground like I did. The height doesn’t matter. I did this on a breezy day, so you’ll have to take my word that it was vertical(ish).
I did this on a breezy day, so you’ll have to take my word that it was vertical(ish). I used the scissors that I cut the string with. I could have also used a rock or a stick or my shoe.
I used the scissors that I cut the string with. I could have also used a rock or a stick or my shoe.

 If you think about it, you are really measuring the angle of the shadow.
If you think about it, you are really measuring the angle of the shadow.
